3周期性边界条件

《3周期性边界条件》由会员分享，可在线阅读，更多相关《3周期性边界条件（29页珍藏版）》请在装配图网上搜索。

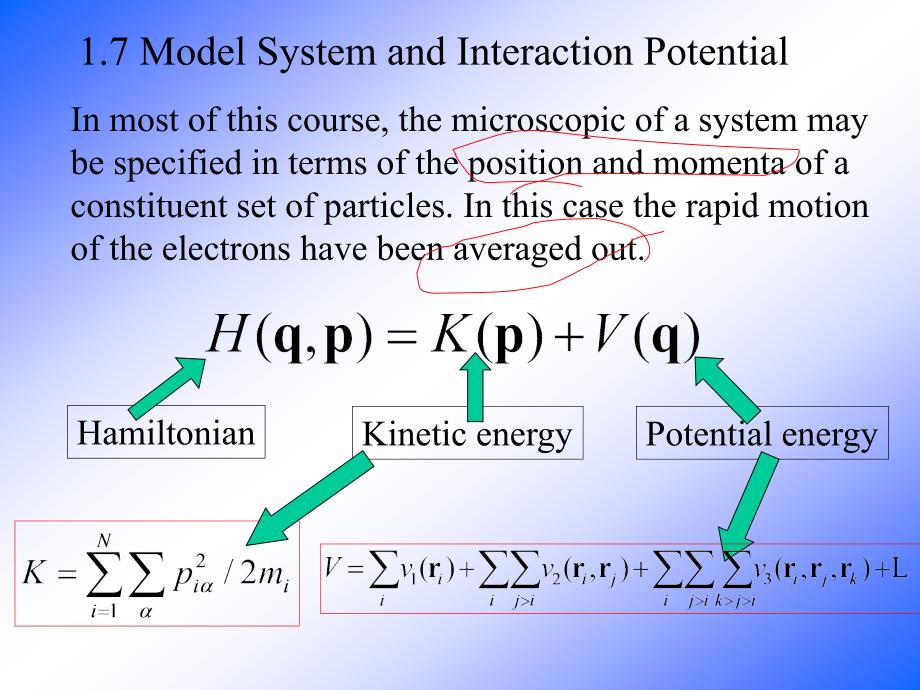
1、单击此处编辑母版标题样式,单击此处编辑母版文本样式,第二级,第三级,第四级,第五级,,第一章 绪论,‹#›,1.7 Model System and Interaction Potential,In most of this course, the microscopic of a system may be specified in terms of the position and momenta of a constituent set of particles. In this case the rapid motion of the electrons have been avera

2、ged out.,,,Hamiltonian,,Kinetic energy,,Potential energy,,,,Analysis of the Potential Energy,,External potential,,Pair potential,about 90%,,Three body contribution,In FCC crystal, up to 10%,,,Effective pair potential,1.7.1 Effective pair potential for spherical molecules,1.7.1.1 Hard-sphere potentia

3、l,For the purposes of investigating general properties of liquids and for comparison with theory, highly idealized pair potentials may be of value. In this section , I will introduce hard-sphere, square-well, Yukawa and Lennard-Jones potentials, etc.,,,,,is the diameter of hard spheres,,,,,,1.7.1.2

4、Square-Well potential,,,,,,,,SW potential is the simplest one including the attractive forces and can be applied to,inert gases and some non-polar substances, etc.,1.7.1.3 Yukawa potential,,When , it can,be used to model Ar reasonably well.,Yukwa potential can also be used to model plas

5、ma, colloidal particles, and some electrical interactions.,,,,1.7.1.4 Lennard-Jones potential,For argon:,Codes for calculating the total potential,V=0.0,DO 100 I=1,N-1,RXI=RX(I),RYI=RY(I),RZI=RZ(I),DO 100 J=I+1,N,RXIJ=RXI-RX(J),RYIJ=RYI-RY(J),RZIJ=RZI-RZ(J),RIJSQ=RXIJ**2+RYIJ**2+RZIJ**2,SR2=SIGSQ/RI

6、JSQ,SR6=SR2*SR2*SR2,SR12=SR6**2,V=V+SR12-SR6,100 CONTINUE,V=V*4.0*EPSLON,The coordinate vectors of LJ atoms are stored in three arrays RX(I), RY(I), RZ(I),,Calculate,,,1.7.1.5 Potentials for ions,,A simple approach to construct potentials for ions is to supplement one of the above pair potentials w

7、ith the Coulomb charge-charge interaction:,Where X may be HS, SW, LJ. The popular one is:,1.7.2 potential for macromolecules,The energy,,V,,is a function of the atomic positions,,R,, of all the atoms in the system, these are usually expressed in term of Cartesian coordinates. The value of the energ

8、y is calculated as a sum of internal, or bonded terms , which describe the bonds, angles and bond rotations in a molecule, and a sum of external or nonbonded terms. These terms account for interactions between nonbonded atoms or atoms separated by 3 or more covalent bonds.,,,Bonded potential,,,Bond

9、angle-bend,,,Bond-strentch,,,Torsion(rotate-along-bond),Angle-bend potential,The deviation of angles from their reference values is frequently described using a Hooke’s law or harmonic potential:,Bond-stretch potential,Torsion potential,Torsional potentials are almost always expressed as a cosine se

10、ries expansion.,,,‘barrier’ height,,,Determines where the torsion angle passes through its minimum value.,,,The number of minimum points in the function as the bond is rotated through 2,.,1.8 Reduced Units,Why use reduced unit?,Avoids the possible embarrassment of conducting essentially,duplicate,s

11、imulations. And there are also technical advantages in the use of reduced units due to the simulation box is in the,magnitude of molecular scale,.,Density,,Temperature,,Energy,,Pressure,,Force,,Torque,,Surface tension,Time,Reduced unit----continue,Diffusion coefficient,,Viscosity,Thermal conductivit

12、y,SI Units:,W/(m K),Pa s,m,2,/s,Test of unit (For example, viscosity):,,,,,,,,,,Reduced unit----continue,It should be pointed that all the reduced units in the previous two slides are based on Lennard-Jones interaction potential. For hard sphere fluid, the reduced units are obtained using,k,B,T,to r

13、eplace,,.,The reduced units for other properties such as chemical potential and heat capacity can be deduced from the units given in this section. Especially, for electrolyte solution, we can use,Surface charge density:,,1.9 Simulation Box and Its Boundary Conditions,Computer simulations are usuall

14、y performed on a,small number of molecules, 10<,N,<10,000. The time,taken for a double loop used to evaluate the forces,and potential energy is proportional to,N,2,.,Whether or not the cube is surrounded by a,containing wall, molecules on the surface will,experience quite different forces from molec

15、ules in,the bulk.,It is essential to propose proper methods to,overcome the problem of surface effects.,1.9.1 Simulation box,,,,,x,y,z,Cube,,,,,,Hexagonal prism,,,,x,y,z,Example:,DNA simulation,1.9.1 Simulation box---continue,Truncated octahedron,Rhombic dodecahedron,1.9.2 Periodic boundary conditio

16、n,,,,,,,,,,B,A,H,D,G,F,E,C,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,B,A,H,D,G,F,E,C,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,In a cubic box, the cutoff distance is set equal to L/2.,,,Minimum image convention,,,,A,E,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,

17、,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,A side view,of the box,(b) A top view,of the box,B,D,C,,,,,,,,,,,,,,,,,A,E,,,,,,,,,,,,,,,,H,F,G,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,Simulation of molecules in slit-like pore,1.9.3 Computer code for periodic boundaries,How do we handle peri

18、odic boundaries and the,minimum image convention in a simulation,program?,Let us assume, initially, the N molecules in the,simulation lie with a cubic box of side,BOXL,,,with the origin at its center, i.e., all coordinate lie,in the range,(-BOXL/2, BOXL/2),. After the,molecules have been moved, we m

19、ust test the,position immediately using a,FORTRAN IF,,statement.,IF(RX(I).GT.BOXL2) RX(I)=RX(I)-BOXL,IF(RX(I).LT.-BOXL2) RX(I)=RX(I)+BOXL,,,,An alternative code for periodic boundaries,An alternative to the IF statement is to use,FORTRAN arithmetic,,functions:,RX(I)=RX(I)-BOXL*ANINT(RX(I)/BOXL),The

20、function,ANINT(X),returns the,nearest integer,to,X,, converting the results back to type,REAL,.,For example,,ANINT(-0.49)=0; ANINT(-0.55)=-1,,The function ANINT(X) is different from AINT(X).,AINT(X) returns the,integral part,of X.,The use of IF statement inside the inner loop,,particularly on pipeli

21、ne machines, is to be avoided.,1.9.4 Computer code for minimum image convention,Immediately after calculating a pair separation vector, we apply the code similar to the periodic boundary adjustments.,RXIJ=RXIJ-BOXL*ANINT(RXIJ/BOXL),RYIJ=RYIJ-BOXL*ANINT(RYIJ/BOXL),RZIJ=RZIJ-BOXL*ANINT(RZIJ/BOXL),If w

22、e use a FORTRAN variable RCUTSQ to represent the square of cutoff distance,r,c,. After the above codes, the following statements would be employed:,RIJSQ=RXIJ**2+RYIJ**2+RZIJ**2,IF(RIJSQ.LT.RCUTSQ)THEN,… compute,i-j,interaction……,… accumulate energy and force…….,,ENDIF,,RIJSQ=RXIJ**2+RYIJ**2+RZIJ**2

23、,RIJSQI=1.0/RIJSQ,RIJSQI=,CVMGP(RIJSQI, 0.0, RCUTSQ-RIJSQ),…compute I-j interaction,….as a functions of RIJSQI….,……,recommended,,,The function,CVMGP(A,B,C),is a vector merge statement which returns to the,value A if C is non-negative,and the value B otherwise.,For example:,,,CVMGP(9, 0, 0)=9,,CVMGP(

24、9, 8, 2)=9,,CVMGP(9, 8, -1)=8,The computer code for other shapes of simulation boxes can be found,,in program F1.,,1.9.5 Non-periodic boundary methods,Periodic boundary conditions are not always used in computer simulation. Why?,Some systems, such as liquid droplets or van der,Waals clusters, inher

25、ently contain a boundary.,When simulating inhomongeneous systems or,systems that are not at equilibrium, periodic,boundary conditions may cause difficulties.,In the study of the structural and conformational,behavior of macromolecules such as proteins and,protein-ligand complexes, the use of periodi

26、c,boundary conditions would require a prohibitive,number of atoms to be included in the simulation.,,,,Example for non-periodic boundary conditions-----study the active site of an enzyme,Reaction zone:,r,<,R,1,.,Containing atoms or,group with the site of,interest. Perform full,simulation.,Reservoir region:,R,1,<,r,<,R,2,,atoms are fixed at initial,position or restrained to,initial positions using a,harmonic potential.,,r,>,R,2,, discarded or fixed.,Division into reaction zone and reservoir regions in a simulation,,,,